Square and Cube Roots of Real Numbers

1.3 Square and Cube Roots of Real Numbers

Learning Objectives

Calculate the exact and approximate value of the square root of a real number.
Calculate the exact and approximate value of the cube root of a real number.
Simplify the square and cube root of a real number.
Apply the Pythagorean theorem.

The Definition of Square and Cube Roots

A square rootThat number that when multiplied by itself yields the original number. of a number is a number that when multiplied by itself yields the original number. For example, 4 is a square root of 16, because $4^{2} = 16 .$ Since ${(- 4)}^{2} = 16$ , we can say that −4 is a square root of 16 as well. Every positive real number has two square roots, one positive and one negative. For this reason, we use the radical signThe symbol $\sqrt{}$ used to denote a square root. $\sqrt{}$ to denote the principal (nonnegative) square rootThe non-negative square root. and a negative sign in front of the radical $- \sqrt{}$ to denote the negative square root.

$\begin{matrix} \sqrt{16} & = & 4 & P o s i t i v e s q u a r e r o o t o f 16 \\ - \sqrt{16} & = & - 4 & N e g a t i v e s q u a r e r o o t o f 16 \end{matrix}$

Zero is the only real number with exactly one square root.

$\sqrt{0} = 0$

If the radicandThe number within a radical., the number inside the radical sign, is nonzero and can be factored as the square of another nonzero number, then the square root of the number is apparent. In this case, we have the following property:

$\begin{matrix} \sqrt{a^{2}} = a, & if & a \geq 0 \end{matrix}$

It is important to point out that a is required to be nonnegative. Note that $\sqrt{{(- 3)}^{2}} \neq - 3$ because the radical denotes the principal square root. Instead,

$\sqrt{{(- 3)}^{2}} = \sqrt{9} = 3$

This distinction will be carefully considered later in the course.

Example 1

Find the square root:

$\sqrt{121}$
$\sqrt{0.25}$
$\sqrt{\frac{4}{9}}$

Solution:

$\sqrt{121} = \sqrt{11^{2}} = 11$
$\sqrt{0.25} = \sqrt{{0.5}^{2}} = 0.5$
$\sqrt{\frac{4}{9}} = \sqrt{{(\frac{2}{3})}^{2}} = \frac{2}{3}$

Example 2

Find the negative square root:

$- \sqrt{64}$
$- \sqrt{1}$

Solution:

$- \sqrt{64} = - \sqrt{8^{2}} = - 8$
$- \sqrt{1} = - \sqrt{1^{2}} = - 1$

The radicand may not always be a perfect square. If a positive integer is not a perfect square, then its square root will be irrational. Consider $\sqrt{5}$ , we can obtain an approximation by bounding it using the perfect squares 4 and 9 as follows:

$\begin{matrix} \sqrt{4} < \sqrt{5} < \sqrt{9} \\ 2 < \sqrt{5} < 3 \end{matrix}$

With this we conclude that $\sqrt{5}$ is somewhere between 2 and 3. This number is better approximated on most calculators using the square root button, $\begin{matrix} \sqrt{} \end{matrix} .$

$\begin{array}{l} \sqrt{5} \approx 2.236 & because & 2.236^2 \approx 5 \end{array}$

Next, consider the square root of a negative number. To determine the square root of −9, you must find a number that when squared results in −9,

$\begin{matrix} \sqrt{- 9} = ? & or & {(?)}^{2} = - 9 \end{matrix}$

However, any real number squared always results in a positive number,

$\begin{matrix} {(3)}^{2} = 9 & and & {(- 3)}^{2} = 9 \end{matrix}$

The square root of a negative number is currently left undefined. Try calculating $\sqrt{- 9}$ on your calculator; what does it say? For now, we will state that $\sqrt{- 9}$ is not a real number. The square root of a negative number is defined later in the course.

A cube rootThe number that when multiplied by itself three times yields the original number, denoted by $\sqrt[3]{} .$ of a number is a number that when multiplied by itself three times yields the original number. Furthermore, we denote a cube root using the symbol $\sqrt[3]{}$ , where 3 is called the indexThe positive integer n in the notation $\sqrt[n]{}$ that is used to indicate an nth root.. For example,

$\begin{matrix} \sqrt[3]{8} = 2, & because & 2^{3} = 8 \end{matrix}$

The product of three equal factors will be positive if the factor is positive, and negative if the factor is negative. For this reason, any real number will have only one real cube root. Hence the technicalities associated with the principal root do not apply. For example,

$\begin{matrix} \sqrt[3]{- 8} = - 2, & because & {(- 2)}^{3} = - 8 \end{matrix}$

In general, given any real number a, we have the following property:

$\sqrt[3]{a^{3}} = a$

When simplifying cube roots, look for factors that are perfect cubes.

Example 3

Find the cube root:

$\sqrt[3]{125}$
$\sqrt[3]{0}$
$\sqrt[3]{\frac{8}{27}}$

Solution:

$\sqrt[3]{125} = \sqrt[3]{5^{3}} = 5$
$\sqrt[3]{0} = \sqrt[3]{0^{3}} = 0$
$\sqrt[3]{\frac{8}{27}} = \sqrt[3]{{(\frac{2}{3})}^{3}} = \frac{2}{3}$

Example 4

Find the cube root:

$\sqrt[3]{- 27}$
$\sqrt[3]{- 1}$

Solution:

$\sqrt[3]{- 27} = \sqrt[3]{{(- 3)}^{3}} = - 3$
$\sqrt[3]{- 1} = \sqrt[3]{{(- 1)}^{3}} = - 1$

It may be the case that the radicand is not a perfect cube. If this is the case, then its cube root will be irrational. For example, $\sqrt[3]{2}$ is an irrational number, which can be approximated on most calculators using the root button $\begin{matrix} \sqrt[x]{} \end{matrix} .$ Depending on the calculator, we typically type in the index prior to pushing the button and then the radicand as follows:

$\begin{matrix} 3 & \begin{matrix} \sqrt[x]{y} \end{matrix} & 2 & \begin{matrix} = \end{matrix} \end{matrix}$

Therefore, we have

$\begin{matrix} \sqrt[3]{2} \approx 1.260, & because & 1.260^3 \approx 2 \end{matrix}$

We will extend these ideas using any integer as an index later in this course. It is important to point out that a square root has index 2; therefore, the following are equivalent:

$\sqrt[2]{a} = \sqrt{a}$

In other words, if no index is given, it is assumed to be the square root.

Simplifying Square and Cube Roots

It will not always be the case that the radicand is a perfect square. If not, we use the following two properties to simplify the expression. Given real numbers $\sqrt[n]{A}$ and $\sqrt[n]{B}$ where $B \neq 0$ ,

Product Rule for Radicals:	$\sqrt[n]{A \cdot B} = \sqrt[n]{A} \cdot \sqrt[n]{B}$
Quotient Rule for Radicals:	$\sqrt[n]{\frac{A}{B}} = \frac{\sqrt[n]{A}}{\sqrt[n]{B}}$

Given real numbers $\sqrt[n]{A}$ and $\sqrt[n]{B}$ , $\sqrt[n]{A \cdot B} = \sqrt[n]{A} \cdot \sqrt[n]{B} .$

Given real numbers $\sqrt[n]{A}$ and $\sqrt[n]{B}$ , $\sqrt[n]{\frac{A}{B}} = \frac{\sqrt[n]{A}}{\sqrt[n]{B}} .$

A simplified radicalA radical where the radicand does not consist of any factors that can be written as perfect powers of the index. is one where the radicand does not consist of any factors that can be written as perfect powers of the index. Given a square root, the idea is to identify the largest square factor of the radicand and then apply the property shown above. As an example, to simplify $\sqrt{12}$ , notice that 12 is not a perfect square. However, 12 does have a perfect square factor, $12 = 4 \cdot 3 .$ Apply the property as follows: $\begin{matrix} \sqrt{12} & = & \sqrt{4 \cdot 3} & A p p l y t h e p r o d u c t r u l e f o r r a d i c a l s . \\ = & \sqrt{4} \cdot \sqrt{3} & S i m p l i f y . \\ = & 2 \cdot \sqrt{3} \end{matrix}$ The number $2 \sqrt{3}$ is a simplified irrational number. You are often asked to find an approximate answer rounded off to a certain decimal place. In that case, use a calculator to find the decimal approximation using either the original problem or the simplified equivalent. $\sqrt{12} = 2 \sqrt{3} \approx 3.46$ As a check, calculate $\sqrt{12}$ and $2 \sqrt{3}$ on a calculator and verify that the results are both approximately 3.46.

Example 5

Simplify: $\sqrt{135} .$

Solution:

Begin by finding the largest perfect square factor of 135.

$\begin{matrix} 135 & = & 3^{3} \cdot 5 \\ = & 3^{2} \cdot 3 \cdot 5 \\ = & 9 \cdot 15 \end{matrix}$

Therefore,

$\begin{matrix} \sqrt{135} & = & \sqrt{9 \cdot 15} & A p p l y t h e p r o d u c t r u l e f o r r a d i c a l s . \\ = & \sqrt{9} \cdot \sqrt{15} & S i m p l i f y . \\ = & 3 \cdot \sqrt{15} \end{matrix}$

Answer: $3 \sqrt{15}$

Example 6

Simplify: $\sqrt{\frac{108}{169}} .$

Solution:

We begin by finding the prime factorizations of both 108 and 169. This will enable us to easily determine the largest perfect square factors.

$\begin{matrix} 108 & = & 2^{2} \cdot 3^{3} = & 2^{2} \cdot 3^{2} \cdot 3 \\ 169 & = & 13^{2} \end{matrix}$

Therefore,

$\begin{matrix} \sqrt{\frac{108}{169}} & = & \sqrt{\frac{2^{2} \cdot 3^{2} \cdot 3}{13^{2}}} & A p p l y t h e p r o d u c t a n d q u o t i e n t r u l e f o r r a d i c a l s . \\ = & \frac{\sqrt{2^{2}} \cdot \sqrt{3^{2}} \cdot \sqrt{3}}{\sqrt{13^{2}}} & S i m p l i f y . \\ = & \frac{2 \cdot 3 \cdot \sqrt{3}}{13} \\ = & \frac{6 \sqrt{3}}{13} \end{matrix}$

Answer: $\frac{6 \sqrt{3}}{13}$

Example 7

Simplify: $- 5 \sqrt{162} .$

Solution:

$\begin{matrix} - 5 \sqrt{162} & = & - 5 \cdot \sqrt{81 \cdot 2} \\ = & - 5 \cdot \sqrt{81} \cdot \sqrt{2} \\ = & - 5 \cdot 9 \cdot \sqrt{2} \\ = & - 45 \cdot \sqrt{2} \\ = & - 45 \sqrt{2} \end{matrix}$

Answer: $- 45 \sqrt{2}$

Try this! Simplify: $4 \sqrt{150} .$

Answer: $20 \sqrt{6}$

(click to see video)

A cube root is simplified if it does not contain any factors that can be written as perfect cubes. The idea is to identify the largest cube factor of the radicand and then apply the product or quotient rule for radicals. As an example, to simplify $\sqrt[3]{80}$ , notice that 80 is not a perfect cube. However, $80 = 8 \cdot 10$ and we can write,

$\begin{matrix} \sqrt[3]{80} & = & \sqrt[3]{8 \cdot 10} & A p p l y t h e p r o d u c t r u l e f o r r a d i c a l s . \\ = & \sqrt[3]{8} \cdot \sqrt[3]{10} & S i m p l i f y . \\ = & 2 \cdot \sqrt[3]{10} \end{matrix}$

Example 8

Simplify: $\sqrt[3]{162} .$

Solution:

Begin by finding the largest perfect cube factor of 162.

$\begin{matrix} 162 & = & 3^{4} \cdot 2 \\ = & 3^{3} \cdot 3 \cdot 2 \\ = & 27 \cdot 6 \end{matrix}$

Therefore,

$\begin{matrix} \sqrt[3]{162} & = & \sqrt[3]{27 \cdot 6} & A p p l y t h e p r o d u c t r u l e f o r r a d i c a l s . \\ = & \sqrt[3]{27} \cdot \sqrt[3]{6} & S i m p l i f y . \\ = & 3 \cdot \sqrt[3]{6} \end{matrix}$

Answer: $3 \sqrt[3]{6}$

Example 9

Simplify: $\sqrt[3]{- \frac{16}{343}} .$

Solution:

$\begin{matrix} \sqrt[3]{- \frac{16}{343}} & = & \frac{\sqrt[3]{- 1 \cdot 8 \cdot 2}}{\sqrt[3]{7^{3}}} \\ = & \frac{\sqrt[3]{- 1} \cdot \sqrt[3]{8} \cdot \sqrt[3]{2}}{\sqrt[3]{7^{3}}} \\ = & \frac{- 1 \cdot 2 \cdot \sqrt[3]{2}}{7} \\ = & \frac{- 2 \sqrt[3]{2}}{7} \end{matrix}$

Answer: $\frac{- 2 \sqrt[3]{2}}{7}$

Try this! Simplify: $- 2 \sqrt[3]{- 256} .$

Answer: $8 \sqrt[3]{4}$

(click to see video)

Consider the following two calculations,

$\begin{matrix} \sqrt{81} & = & \sqrt{9^{2}} = 9 \\ \sqrt{81} & = & \sqrt{9^{2}} = {(\sqrt{9})}^{2} = {(3)}^{2} = 9 \end{matrix}$

Notice that it does not matter if we apply the exponent first or the square root first. This is true for any positive real number. We have the following,

$\begin{matrix} \sqrt{a^{2}} = {(\sqrt{a})}^{2} = a, & if & a \geq 0 \end{matrix}$

Example 10

Simplify: ${(\sqrt{10})}^{2} .$

Solution:

Apply the fact that ${(\sqrt{a})}^{2} = a$ if a is nonnegative.

${(\sqrt{10})}^{2} = 10$

Pythagorean Theorem

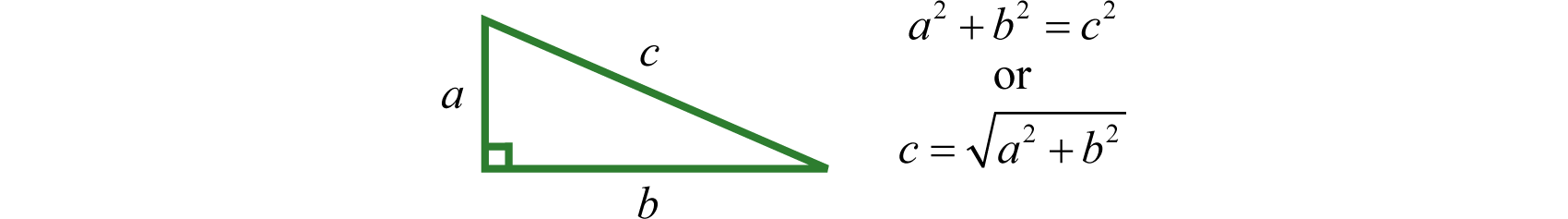
A right triangleA triangle with an angle that measures 90°. is a triangle where one of the angles measures 90°. The side opposite the right angle is the longest side, called the hypotenuseThe longest side of a right triangle; it will always be the side opposite the right angle., and the other two sides are called legsThe sides of a right triangle that are not the hypotenuse.. Numerous real-world applications involve this geometric figure. The Pythagorean theoremThe hypotenuse of any right triangle is equal to the square root of the sum of the squares of the lengths of the triangle’s legs. states that given any right triangle with legs measuring a and b units, the square of the measure of the hypotenuse c is equal to the sum of the squares of the measures of the legs, $a^{2} + b^{2} = c^{2} .$ In other words, the hypotenuse of any right triangle is equal to the square root of the sum of the squares of its legs.

Example 11

Calculate the diagonal of a square with sides measuring 5 units.

Solution:

The diagonal of a square will form an isosceles right triangle where the two equal legs measure 5 units each.

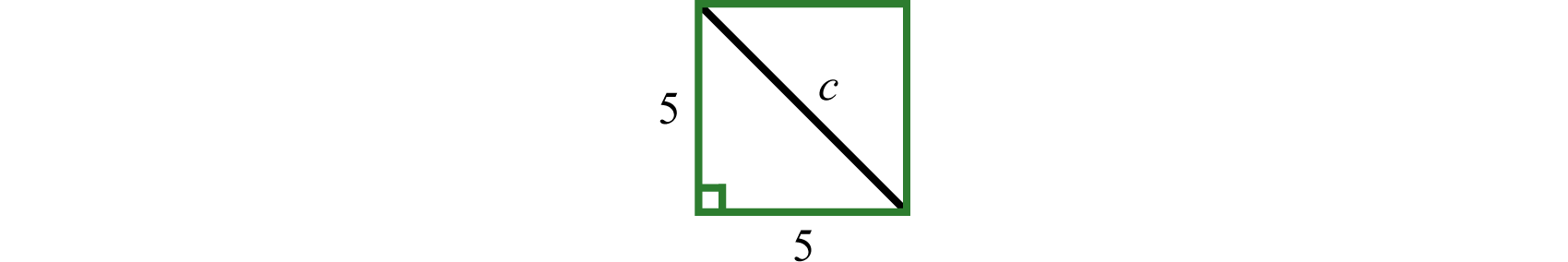
We can use the Pythagorean theorem to determine the length of the hypotenuse.

$\begin{matrix} c & = & \sqrt{a^{2} + b^{2}} \\ = & \sqrt{5^{2} + 5^{2}} \\ = & \sqrt{25 + 25} \\ = & \sqrt{50} \\ = & \sqrt{25 \cdot 2} \\ = & \sqrt{25} \cdot \sqrt{2} \\ = & 5 \cdot \sqrt{2} \end{matrix}$

Answer: $5 \sqrt{2}$ units

The Pythagorean theorem actually states that having side lengths satisfying the property $a^{2} + b^{2} = c^{2}$ is a necessary and sufficient condition of right triangles. In other words, if we can show that the sum of the squares of the lengths of the legs of the triangle is equal to the square of the hypotenuse, then it must be a right triangle.

Example 12

Determine whether or not a triangle with legs $a = 1$ cm and $b = 2$ cm and hypotenuse $b = \sqrt{5}$ cm is a right triangle.

Solution:

If the legs satisfy the condition $a^{2} + b^{2} = c^{2}$ then the Pythagorean theorem guarantees that the triangle is a right triangle.

$\begin{matrix} a^{2} + b^{2} & = & c^{2} \\ {(1)}^{2} + {(2)}^{2} & \overset{?}{=} & {(\sqrt{5})}^{2} \\ 1 + 4 & = & 5 \\ 5 & = & 5 & ✓ \end{matrix}$

Answer: Yes, the described triangle is a right triangle.

Key Takeaways

The square root of a number is a number that when squared results in the original number. The principal square root of a positive real number is the positive square root. The square root of a negative number is currently left undefined.
When simplifying the square root of a number, look for perfect square factors of the radicand. Apply the product or quotient rule for radicals and then simplify.
The cube root of a number is a number that when cubed results in the original number. Every real number has only one real cube root.
When simplifying cube roots, look for perfect cube factors of the radicand. Apply the product or quotient rule for radicals and then simplify.
The Pythagorean theorem gives us a necessary and sufficient condition of right triangles: $a^{2} + b^{2} = c^{2}$ if and only if a, b and c represent the lengths of the sides of a right triangle.

Topic Exercises

Part A: Square and Cube Roots

Simplify.

$\sqrt{81}$
$\sqrt{49}$
$- \sqrt{16}$
$- \sqrt{100}$
$\sqrt{\frac{25}{16}}$
$\sqrt{\frac{9}{64}}$
$\sqrt{\frac{1}{4}}$
$\sqrt{\frac{1}{100}}$
$\sqrt{- 1}$
$\sqrt{- 25}$
$\sqrt{0.36}$
$\sqrt{1.21}$
$\sqrt{{(- 5)}^{2}}$
$\sqrt{{(- 6)}^{2}}$
$2 \sqrt{64}$
$3 \sqrt{36}$
$- 10 \sqrt{4}$
$- 8 \sqrt{25}$
$\sqrt[3]{64}$
$\sqrt[3]{125}$
$\sqrt[3]{- 27}$
$\sqrt[3]{- 1}$
$\sqrt[3]{0}$
$\sqrt[3]{0.008}$
$\sqrt[3]{0.064}$
$- \sqrt[3]{- 8}$
$- \sqrt[3]{1000}$
$\sqrt[3]{{(- 8)}^{3}}$
$\sqrt[3]{{(- 15)}^{3}}$
$\sqrt[3]{\frac{1}{216}}$
$\sqrt[3]{\frac{27}{64}}$
$\sqrt[3]{- \frac{1}{8}}$
$\sqrt[3]{- \frac{1}{27}}$
$5 \sqrt[3]{343}$
$4 \sqrt[3]{512}$
$- 10 \sqrt[3]{8}$
$- 6 \sqrt[3]{- 64}$
$8 \sqrt[3]{- 8}$

Use a calculator to approximate to the nearest hundredth.

$\sqrt{3}$
$\sqrt{10}$
$\sqrt{19}$
$\sqrt{7}$
$3 \sqrt{5}$
$- 2 \sqrt{3}$
$\sqrt[3]{3}$
$\sqrt[3]{6}$
$\sqrt[3]{28}$
$\sqrt[3]{9}$
$4 \sqrt[3]{10}$
$- 3 \sqrt[3]{12}$
Determine the set consisting of the squares of the first twelve positive integers.
Determine the set consisting of the cubes of the first twelve positive integers.

Part B: Simplifying Square Roots and Cube Roots

Simplify.

$\sqrt{18}$
$\sqrt{50}$
$\sqrt{24}$
$\sqrt{40}$
$\sqrt{\frac{50}{81}}$
$\sqrt{\frac{54}{25}}$
$4 \sqrt{72}$
$3 \sqrt{27}$
$- 5 \sqrt{80}$
$- 6 \sqrt{128}$
$3 \sqrt{- 40}$
$5 \sqrt{- 160}$
$\sqrt[3]{16}$
$\sqrt[3]{54}$
$\sqrt[3]{81}$
$\sqrt[3]{24}$
$\sqrt[3]{\frac{48}{125}}$
$\sqrt[3]{\frac{135}{64}}$
$7 \sqrt[3]{500}$
$25 \sqrt[3]{686}$
$- 2 \sqrt[3]{- 162}$
$5 \sqrt[3]{- 96}$
${(\sqrt{64})}^{2}$
${(\sqrt{25})}^{2}$
${(\sqrt{2})}^{2}$
${(\sqrt{6})}^{2}$

Part C: Pythagorean Theorem

If the two legs of a right triangle measure 3 units and 4 units, then find the length of the hypotenuse.
If the two legs of a right triangle measure 6 units and 8 units, then find the length of the hypotenuse.
If the two equal legs of an isosceles right triangle measure 7 units, then find the length of the hypotenuse.
If the two equal legs of an isosceles right triangle measure 10 units, then find the length of the hypotenuse.
Calculate the diagonal of a square with sides measuring 3 centimeters.
Calculate the diagonal of a square with sides measuring 10 centimeters.
Calculate the diagonal of a square with sides measuring $\sqrt{6}$ centimeters.
Calculate the diagonal of a square with sides measuring $\sqrt{10}$ centimeters.
Calculate the length of the diagonal of a rectangle with dimensions 4 centimeters by 8 centimeters.
Calculate the length of the diagonal of a rectangle with dimensions 8 meters by 10 meters.
Calculate the length of the diagonal of a rectangle with dimensions $\sqrt{3}$ meters by 2 meters.
Calculate the length of the diagonal of a rectangle with dimensions $\sqrt{6}$ meters by $\sqrt{10}$ meters.
To ensure that a newly built gate is square, the measured diagonal must match the distance calculated using the Pythagorean theorem. If the gate measures 4 feet by 4 feet, what must the diagonal measure in inches? (Round off to the nearest tenth of an inch.)
If a doorframe measures 3.5 feet by 6.6 feet, what must the diagonal measure to ensure that the frame is a perfect rectangle?

Determine whether or not the given triangle with legs a and b and hypotenuse c is a right triangle or not.

$a = 3$ , $b = 7$ , and $c = 10$
$a = 5$ , $b = 12$ , and $c = 13$
$a = 8$ , $b = 15$ , and $c = 17$
$a = 7$ , $b = 24$ , and $c = 30$
$a = 3$ , $b = 2$ , and $c = \sqrt{13}$
$a = \sqrt{7}$ , $b = 4$ , and $c = \sqrt{11}$
$a = 4$ , $b = \sqrt{3}$ , and $c = \sqrt{19}$
$a = \sqrt{6}$ , $b = \sqrt{15}$ , and $c = \sqrt{21}$

Part D: Discussion Board

What does your calculator say after taking the square root of a negative number? Share your results on the discussion board and explain why it says that.
Research and discuss the history of the Pythagorean theorem.
Research and discuss the history of the square root.
Discuss the importance of the principal square root. Why is it that the same issue does not come up with cube roots? Provide some examples with your explanation.

Answers

9
−4
$\frac{5}{4}$
$\frac{1}{2}$
Not a real number.
0.6
5
16
−20
4
−3
0
0.4
−10
−15
$\frac{3}{4}$
$- \frac{1}{3}$
32
24
1.73
4.36
6.71
1.44
3.04
8.62
{1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144}

$3 \sqrt{2}$
$2 \sqrt{6}$
$\frac{5 \sqrt{2}}{9}$
$24 \sqrt{2}$
$- 20 \sqrt{5}$
Not a real number.
$2 \sqrt[3]{2}$
$3 \sqrt[3]{3}$
$\frac{2 \sqrt[3]{6}}{5}$
$35 \sqrt[3]{4}$
$6 \sqrt[3]{6}$
64
2

5 units
$7 \sqrt{2}$ units
$3 \sqrt{2}$ centimeters
$2 \sqrt{3}$ centimeters
$4 \sqrt{5}$ centimeters
$\sqrt{7}$ meters
The diagonal must measure approximately 67.9 inches.
Not a right triangle.
Right triangle.
Right triangle.
Right triangle.

Answer may vary
Answer may vary