Response surface model

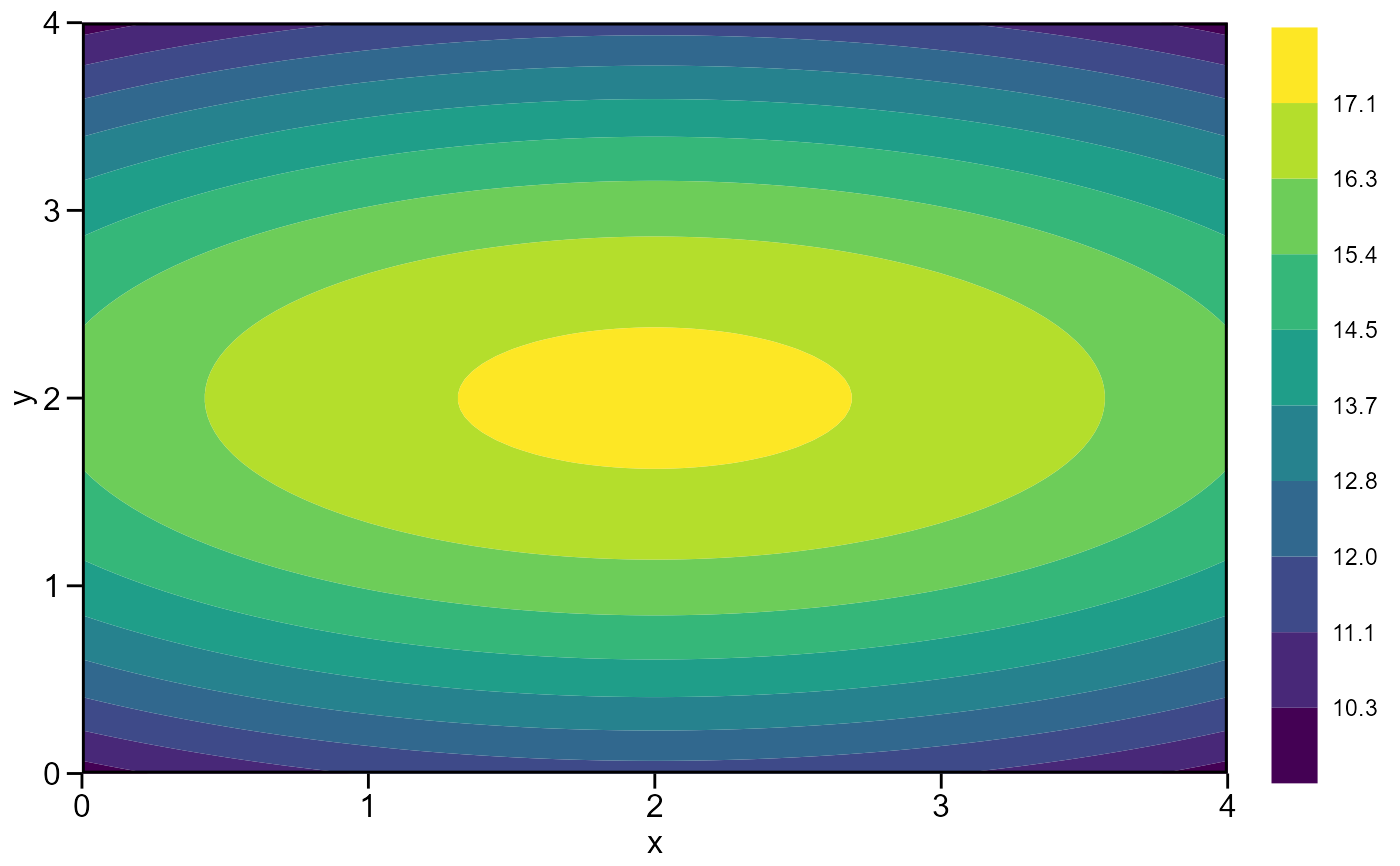
Compute a surface model and find the best combination of factor1 and factor2 to obtain the stationary point.

Usage

resp_surf(
  .data,
  factor1,
  factor2,
  rep = NULL,
  resp,
  prob = 0.05,
  verbose = TRUE
)

Arguments

.data

The dataset containing the columns related to Environments, factor1, factor2, replication/block and response variable(s).

factor1

The first factor, for example, dose of Nitrogen.

factor2

The second factor, for example, dose of potassium.

rep

The name of the column that contains the levels of the replications/blocks, if a designed experiment was conducted. Defaults to NULL.

resp

The response variable(s).

prob

The probability error.

verbose

If verbose = TRUE then some results are shown in the console.

Author

Tiago Olivoto tiagoolivoto@gmail.com

Examples

# \donttest{
library(metan)
# A small toy example

df <- data.frame(
 expand.grid(x = seq(0, 4, by = 1),
             y = seq(0, 4, by = 1)),
 z = c(10, 11, 12, 11, 10,
       14, 15, 16, 15, 14,
       16, 17, 18, 17, 16,
       14, 15, 16, 15, 14,
       10, 11, 12, 11, 10)
)
mod <- resp_surf(df, x, y, resp = z)
#> -----------------------------------------------------------------
#> Anova table for the response surface model 
#> -----------------------------------------------------------------
#> Analysis of Variance Table
#> 
#> Response: z
#>           Df  Sum Sq Mean Sq F value    Pr(>F)    
#> x          1   0.000   0.000    0.00         1    
#> y          1   0.000   0.000    0.00         1    
#> I(x^2)     1  12.857  12.857  106.88 3.073e-09 ***
#> I(y^2)     1 142.857 142.857 1187.50 < 2.2e-16 ***
#> x:y        1   0.000   0.000    0.00         1    
#> Residuals 19   2.286   0.120                      
#> ---
#> Signif. codes:  0 '***' 0.001 '**' 0.01 '*' 0.05 '.' 0.1 ' ' 1
#> -----------------------------------------------------------------
#> Model equation for response surface model 
#> Y = B0 + B1*A + B2*D + B3*A^2 + B4*D^2 + B5*A*D 
#> -----------------------------------------------------------------
#> Estimated parameters 
#> B0: 9.8857143
#> B1: 1.7142857
#> B2: 5.7142857
#> B3: -0.4285714
#> B4: -1.4285714
#> B5: 0.0000000
#> -----------------------------------------------------------------
#> Matrix of parameters (A) 
#> -----------------------------------------------------------------
#> -0.4285714    0.0000000 
#> 0.0000000    -1.4285714 
#> -----------------------------------------------------------------
#> Inverse of the matrix A (invA) 
#> -2.3333333    0.0000000 
#> 0.0000000    -0.7000000 
#> -----------------------------------------------------------------
#> Vetor of parameters B1 e B2 (X) 
#> -----------------------------------------------------------------
#> B1: 1.7142857
#> B2: 5.7142857
#> -----------------------------------------------------------------
#> Equation for the optimal points (A and D) 
#> -----------------------------------------------------------------
#> -0.5*(invA*X)
#> Eigenvalue 1: -0.428571
#> Eigenvalue 2: -1.428571
#> Stacionary point is maximum!
#> -----------------------------------------------------------------
#> Stacionary point obtained with the following original units: 
#> -----------------------------------------------------------------
#> Optimal dose (x): 2
#> Optimal dose (y): 2
#> Predicted: 17.3143
#> -----------------------------------------------------------------
#> Fitted model 
#> -----------------------------------------------------------------
#> A = x
#> D = y
#> y = 9.88571+1.71429A+5.71429D+-0.42857A^2+-1.42857D^2+0A*D
#> -----------------------------------------------------------------
#> Shapiro-Wilk normality test
#> p-value:  0.04213785 
#> WARNING: at 5% of significance, residuals can not be considered normal! 
#> ------------------------------------------------------------------
plot(mod)

# }